Field (physics)


In physics, a field is a physical quantity associated to each point of spacetime.[1] A field can be classified as a scalar field, a vector field, a tensor field,or a spinor field according to whether the value of the field at each point is a scalar, a vector, a spinor (e.g., a Dirac electron) or, more generally, a tensor, respectively. For example, the Newtonian gravitational field is a vector field: specifying its value at a point in spacetime requires three numbers, the components of the gravitational field vector at that point. Moreover, within each category (scalar, vector, tensor), a field can be either a classical field or a quantum field, depending on whether it is characterized by numbers or quantum operators respectively.
A field may be thought of as extending throughout the whole of space. In practice, the strength of every known field has been found to diminish to the point of being undetectable. For instance, in Newton's theory of gravity, the gravitational field strength is inversely proportional to the square of the distance from the gravitating object. Therefore the Earth's gravitational field quickly becomes undetectable (on cosmic scales).
Defining the field as "numbers in space" shouldn't detract from the idea that it has physical reality. “It occupies space. It contains energy. Its presence eliminates a true vacuum.”[2] The vacuum is free of matter, but not free of field. The field creates a "condition in space"”[3]
If an electrical charge is moved, the effects on another charge do not appear instantaneously. The first charge feels a reaction force, picking up momentum, but the second charge feels nothing until the influence, traveling at the speed of light, reaches it and gives it the momentum. Where is the momentum before the second charge moves? By the law of conservation of momentum it must be somewhere. Physicists have found it of "great utility for the analysis of forces"[3] to think of it as being in the field.
This utility leads to physicists believing that electromagnetic fields actually exist, making the field concept a supporting paradigm of the entire edifice of modern physics. That said, John Wheeler and Richard Feynman have entertained Newton's pre-field concept of action at a distance (although they put it on the back burner because of the ongoing utility of the field concept for research in general relativity and quantum electrodynamics).
"The fact that the electromagnetic field can possess momentum and energy makes it very real... a particle makes a field, and a field acts on another particle, and the field has such familiar properties as energy content and momentum, just as particles can have"[3].
Contents |
Field theory
Field theory usually refers to a construction of the dynamics of a field, i.e. a specification of how a field changes with time or with respect to other components of the field. Usually this is done by writing a Lagrangian or a Hamiltonian of the field, and treating it as the classical mechanics (or quantum mechanics) of a system with an infinite number of degrees of freedom. The resulting field theories are referred to as classical or quantum field theories.
In modern physics, the most often studied fields are those that model the four fundamental forces which one day may lead to the Unified Field Theory.
Classical fields
There are several examples of classical fields. The dynamics of a classical field are usually specified by the Lagrangian density in terms of the field components; the dynamics can be obtained by using the action principle.
Michael Faraday first realized the importance of a field as a physical object, during his investigations into magnetism. He realized that electric and magnetic fields are not only fields of force which dictate the motion of particles, but also have an independent physical reality because they carry energy.
These ideas eventually led to the creation, by James Clerk Maxwell, of the first unified field theory in physics with the introduction of equations for the electromagnetic field. The modern version of these equations are called Maxwell's equations. At the end of the 19th century, the electromagnetic field was understood as a collection of two vector fields in space. Nowadays, one recognizes this as a single antisymmetric 2nd-rank tensor field in spacetime.
Einstein's theory of gravity, called general relativity, is another example of a field theory. Here the principal field is the metric tensor, a symmetric 2nd-rank tensor field in spacetime.
In a general setting, classical fields are described by sections of fiber bundles and their dynamics is formulated in the terms of jet manifolds (covariant classical field theory).[4]
In BRST theory one deals with odd fields, e.g. ghosts. There are different descriptions of odd classical fields both on graded manifolds and supermanifolds.
Quantum fields
It is now believed that quantum mechanics should underlie all physical phenomena, so that a classical field theory should, at least in principle, permit a recasting in quantum mechanical terms; success yields the corresponding quantum field theory. For example, quantizing classical electrodynamics gives quantum electrodynamics. Quantum electrodynamics is arguably the most successful scientific theory; experimental data confirm its predictions to a higher precision (to more significant digits) than any other theory.[5] The two other fundamental quantum field theories are quantum chromodynamics and the electroweak theory. These three quantum field theories can all be derived as special cases of the so-called standard model of particle physics. General relativity, the classical field theory of gravity, has yet to be successfully quantized.
Classical field theories remain useful wherever quantum properties do not arise, and can be active areas of research. Elasticity of materials, fluid dynamics and Maxwell's equations are cases in point.
Continuous random fields
Classical fields as above, such as the electromagnetic field, are usually infinitely differentiable functions, but they are in any case almost always twice differentiable. In contrast, generalized functions are not continuous. When dealing carefully with classical fields at finite temperature, the mathematical methods of continuous random fields have to be used, because a thermally fluctuating classical field is nowhere differentiable. Random fields are indexed sets of random variables; a continuous random field is a random field that has a set of functions as its index set. In particular, it is often mathematically convenient to take a continuous random field to have a Schwartz space of functions as its index set, in which case the continuous random field is a tempered distribution.
As a (very) rough way to think about continuous random fields, we can think of it as an ordinary function that is 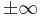 almost everywhere, but when we take a weighted average of all the infinities over any finite region, we get a finite result. The infinities are not well-defined; but the finite values can be associated with the functions used as the weight functions to get the finite values, and that can be well-defined. We can define a continuous random field well enough as a linear map from a space of functions into the real numbers.
almost everywhere, but when we take a weighted average of all the infinities over any finite region, we get a finite result. The infinities are not well-defined; but the finite values can be associated with the functions used as the weight functions to get the finite values, and that can be well-defined. We can define a continuous random field well enough as a linear map from a space of functions into the real numbers.
Symmetries of fields
A convenient way of classifying a field (classical or quantum) is by the symmetries it possesses. Physical symmetries are usually of two types:
Spacetime symmetries
Fields are often classified by their behaviour under transformations of spacetime. The terms used in this classification are —
- scalar fields (such as temperature) whose values are given by a single variable at each point of space. This value does not change under transformations of space.
- vector fields (such as the magnitude and direction of the force at each point in a magnetic field) which are specified by attaching a vector to each point of space. The components of this vector transform between themselves as usual under rotations in space.
- tensor fields, (such as the stress tensor of a crystal) specified by a tensor at each point of space. The components of the tensor transform between themselves as usual under rotations in space.
- spinor fields are useful in quantum field theory.
Internal symmetries
Fields may have internal symmetries in addition to spacetime symmetries. For example, in many situations one needs fields which are a list of space-time scalars: (φ1,φ2...φN). For example, in weather prediction these may be temperature, pressure, humidity, etc. In particle physics, the color symmetry of the interaction of quarks is an example of an internal symmetry of the strong interaction, as is the isospin or flavour symmetry.
If there is a symmetry of the problem, not involving spacetime, under which these components transform into each other, then this set of symmetries is called an internal symmetry. One may also make a classification of the charges of the fields under internal symmetries.
See also
- Elasticity
- Electromagnetic field
- Fluid dynamics
- Gauge theory
- General relativity
- Maxwell's equations
- Particle physics
- Quantum field theory
- Covariant classical field theory
- Standard model
- Symmetry in physics
- Scalar field theory
Notes
- ↑ John Gribbin (1998). Q is for Quantum: Particle Physics from A to Z. London: Weidenfeld & Nicolson. p. 138. ISBN 0297817523.
- ↑ John Archibald Wheeler (1998). Geons, Black Holes, and Quantum Foam: A Life in Physics.. London: Norton. p. 163.
- ↑ 3.0 3.1 3.2 Richard P. Feynman (1963). Feynman's Lectures on Physics, Volume 1.. Caltech. p. 2–4. so that when we put a particle in it it feels a force.
- ↑ Giachetta, G., Mangiarotti, L., Sardanashvily, G. (2009) Advanced Classical Field Theory. Singapore: World Scientific, ISBN 9789812838957 (arXiv: 0811.0331v2)
- ↑ Peskin & Schroeder 1995, p. 198. Also see precision tests of QED.
References
- Landau, Lev D. and Lifshitz, Evgeny M. (1971). Classical Theory of Fields (3rd ed.). London: Pergamon. ISBN 0-08-016019-0. Vol. 2 of the Course of Theoretical Physics.
- Peskin, Michael E.; Schroeder, Daniel V. (1995). An Introduction to Quantum Fields. Westview Press. ISBN 0-201-50397-2.